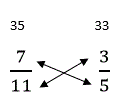A ratio is a relation in which one quantity bears to another(of same kind) with respect to magnitude,the comparison being made by considering what multiple or what part is one quantity to the another.Thus ratio of two quantities is equivalent to the fraction that one quantity is of the other.The ratio between 'P' and 'Q' is P/Q, written as P:Q.P (numerator) is called antecedent and the Q(denominator) is called consequent.Here we are sharing some important formulas and rules for solving ratio and proportion problems.
Ratio and proportion-Important rules and shortcuts
Example:If P:Q=2:3, Q:R=4:5 then P:R=?
P/R=(P/Q)*(Q/R)=2/3*4/5=8/15,thus P:R=8:15
Example:P:Q:R=2:3:4,then P/Q:Q/R:R/P=?
P/Q:Q/R:R/P=2/3:3/4:4/2=8:9:24
Example:If 2P=3Q=4R then P:Q:R=?
Let 2P=3Q=4R=K,
we get P=K/2,Q=K/3,R=K/4
=> P:Q:R=K/2:K/3:K/4=1/2:1/3:1/4=6:4:3
we get P=K/2,Q=K/3,R=K/4
=> P:Q:R=K/2:K/3:K/4=1/2:1/3:1/4=6:4:3
Example:P:Q=1:2,Q:R=4:5,R:S=10:3 then P:Q:R:S=?
Make the Q term in first and second fraction same and make the R term similar in second and third fractions as follows
P:Q:R:S=2:4:5:3/2=4:8:10:3
Comparison of ratios and Fractions
Method1:To compare two fractions we can make either denominators same or numerators same.
Example:2/5 and 3/10
To find out which is greater, make denominators same.We get 4/10 and 3/10.From this we can conclude,2/5>3/10
or make numerator same
Fractions will become 6/15 and 6/20,obviously 6/15>6/20.
Method2:This method can be applied if difference between numerator and denominator is same for all given fractions.
Example:1/2,3/4,7/8. Here 2-1=4-3=8-7=1.In such cases, just look at the numerator .Smaller the numerator will be smaller fraction.1/2<3/4<7/8.
Method3:this method is applicable for all fractions.
If a/b and c/d are fractions under consideration,cross multiply numerator and denominator .ie a*d and c*b.
If a*d>b*c,then a/b>c/d
Example:7/11 and 3/5
cross multiply denominator and numerator.We get 7*5 and 11*3
Since 7*5>11*3 ,7/11>3/5
Ratio and proportion practice questions
Proportion
The equality of the two ratios is called proportion.Suppose the two ratios A:B and C:D are equal,ie A:B=C:D, the we write A:B::C:D
Here A and D are called extremes and B and C are known as means.
AD=BC ,ie product of means=product of extremes.Ratio and proportion practice questions